February 24, 2019 / cpstobart / 0 Comments
It does not matter how we describe it: mathematics learning difficulties; digit dyslexia; arithmetic learning disabilities… there is a phenomenon affecting an estimated 5% of the population. They have difficulty learning and remembering arithmetic facts. They have problems solving problems that involve calculation. The problem these people encounter with numbers is often masked by another difficulty – for example dyslexia.
Dr Josef Gerstmann first started to investigate and write about the difficulties experienced in learning or comprehending mathematics during the 1940s and the term dyscalculia, meaning ‘counting badly’, was coined about 1949. However, it wasn’t until the mid 1970s that, through the work of Ladislav Kosc, dyscalculia was defined as ‘a structural disorder of mathematical abilities.’
What is dyscalculia? Well, there is a wealth of information out there explaining what it is (and isn’t). Good places to start might be the British Dyslexia Association whose website has a section for dyscalculia – it is widely acknowledged that many dyslexics do have dyscalculia. It is worth noting that there is no causal connection between these two. There is, however, a strong connection between students displaying dyscalculia and (maths) anxiety – this should not come as a surprise. Look also at dyscalculia specific organisations such as DyslexiaScotland.org.uk or the Dyscalculia Information Centre.
As a mathematics teacher I need to recognise that I will come across my share of dyscalculics. Just because a student seems distant, anxious or even lazy, has poor attention, or just generally bad a maths, does not mean that they are dyscalculics – but these traits might mask dyscalculia, some of them may be learned ways that the student copes with their difficulty. It means of course that I have to be very sensitive to these students’ needs and to quietly investigate their computational and processing skills.
Dyscalculia cannot be cured, you do not grow out of it. But, it can be managed, skill sets can be improved, strategies can be learned, attention and working memory can be developed, anxiety can be relieved. It is my job to ensure that students are given back their sense of number, to correct a poor concept of number, to start to acquire in a concrete way those foundation blocks that all other concepts are built upon.
This is not, cannot be, a quick fix. Intervention is not about helping out with homework or repeating lessons on a 1 to 1 basis with a student. It is about identifying the mathematical connections that the student does not have, working to build factual blocks that will allow more cumulative skills to be learned. It could be slow – it will depend when intervention starts.
As I build my awareness of this learning difficulty, my experience of working in 1 to 1 relationship with dyscalculics, and evaluate the success of my intervention, I aim to post occasional updates on my reflections and discoveries.
June 29, 2018 / cpstobart / 0 Comments
Past, practice and specimen papers are done by all of us. It is a tried and tested means of getting students used to question style, wording, topic mixture and many other aspects of the exam. Sometimes I feel that we can give students past paper exhaustion – that they are ‘past-papered-out’ by the time the actual exam comes around.
This year we tried to look at how we could use some of last year’s papers in a more structured and reflective way. In April we decide to use one of them as a mock exam (and as a reality check) but to analyse the results in a different way.
I am an active Twitter user (@cpstobart on education related topics only) and there are a number of inspirational maths leaders and teachers out there. Via weekly Twitter conversations at #mathscpdchat and #mathschat, which I join in when I can constructively contribute, ideas are suggested and developed.
I was particularly interested in one idea which required recording the mark gained for every part question for every student. Putting these into a spreadsheet which is colour coded for the marks gained, presented an amazing map of accuracy and understanding.
Here is a portion of the ‘map’:
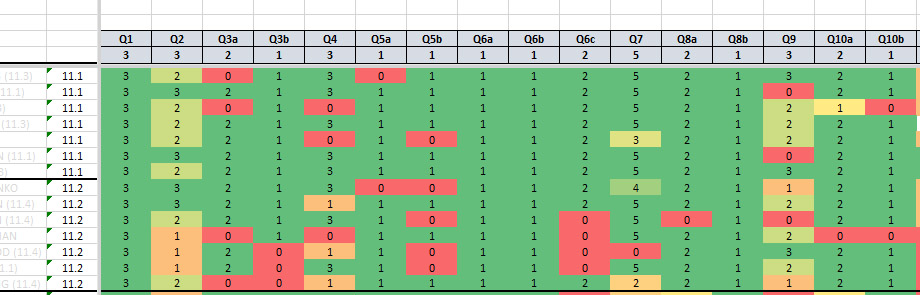
The header rows tell you the Question number and the marks available. Each following row is the set of marks gained by each student, and each column a part question. Colours range from green (full marks) to red (no marks).
The question for us is, What can we do with this information?
We can identify which questions were done well by virtually all students (topics do not need revision) and some which were done poorly by many students (intervention required). We could also see where students had started a question successfully but then did not manage to follow through to a second or third part – why not?
Initially we had thought that we could rearrange students into new groups where students that had the same problem topic could come together for a couple of lessons, then rearrange again, and again. This would provide better managed and targeted revision.
Although this would have been a very good exercise what we discovered was that there were a few part questions where everyone performed poorly. This meant we could keep the classes as normal and tackle the same problem in every group.
The difficulties arose from interpreting and decoding questions successfully. On closer inspection we discovered questions where particular wording had been the problem.
This could have been an unusual context that the question was set in, specific mathematical vocabulary that is muddled, or a cultural misunderstanding. It was surprising how widespread some of the problems turned out to be – but we would not have been able to identify them without this expansive overview.
In many instances, once vocabulary issues were rectified solutions were then successfully found without any further help needed. This does highlight, for us, the importance of vocabulary and context. While we do make extensive use of student word banks we can never relax our efforts to ensure that they are regularly updated. For many students, just a small amount of help resulted in big rewards because a question was suddenly unlocked.
Was this a useful exercise? Without doubt. We had a preconceived idea about what we were going to do but the data took us in another direction along a route that was, ultimately, better than our original idea.
Would we do this again? Absolutely. Having the overall map of student achievement by part question is a terrific snapshot of their levels of understanding. It offers suggestions about the type of intervention that can be usefully employed to clear up misconceptions and deepen understanding.
December 10, 2016 / cpstobart / 0 Comments
In the UKEdMag of October 2016 Kara Collins (@karadubai28) wrote a short and thoughtful article titled: ‘I’m rubbish at Maths’ How personal experience can influence teaching.
This is very honest reflection of early experience when faced with a maths challenge. Is this a kind of maths ‘anxiety’ or phobia? Where does it come from? How does it become such a difficulty?
An AHT once said to me, when discussing a colleague, ‘There are people who teach maths and there are mathematicians.’ The person we were talking about was indeed a mathematician of the highest calibre, but it also made me reflect on my own experience. I am a definitely a ‘maths teacher’ and not a mathematician, but I have a love for maths and the challenges that finding solutions present.
‘I’m rubbish at maths,’ is commonly heard in secondary schools. I say schools rather than classrooms because teachers are guilty of uttering this phrase as well. However, the same can be heard at home as well. As Babtie and Emerson report in their book ‘Understanding Dyscalcula…’ (2015, pg 55-56):
In many western countries there is a tacit anti-maths view. Failure in mathematics is deemed acceptable in adulthood. Parents, and some teachers, will make remarks such as: ‘I was always bad at maths.’ ‘This is really hard.’ The remark is often accompanied by a laugh. Often this is in the context of basic numeracy in the first few years of school. As a result children may receive confusing signals. The subliminal message is that maths is so hard even mum and dad find it difficult, and maths doesn’t really matter.
And this is the difficulty we, as teachers of maths, encounter.
Maths anxiety is defined as a feeling of tension and worry that interferes with the manipulation of numbers and the solving of mathematical problems in everyday situations – not just in the classroom. It’s a debilitating emotional reaction to solving problems involving numeracy.
There is not a single, or isolated, setting where this originates. As suggested above, a student’s family and educational situation can contribute, and when additional factors (such as poverty, access to education – particularly at a young age) surrounding these are considered, it is no wonder that we are not only presented with students who struggle with basic mathematical concepts and processes, but also ones that are turned off maths. The off-switch may well have been flicked by no other reason than the weight of an opinion by a peer, parent, significant other…
The old wives’ tale, or a much-retweeted factoid, that if you say (or hear) something often enough you will start to believe it applies here. What’s known as the Illusory Truth Effect is the idea that if you repeat something often enough, people will slowly start to believe it’s true. And the effect is much stronger than we imagine. The steady drip, drip, drip that maths is difficult, doesn’t matter… will eventually convince perfectly capable student that this is, in fact the case.
How do we combat this? A number of times I have tweeted about culture in the classroom, about the positives of peer-to-peer work, of in-class marking and feedback, of the need to constantly build numeracy confidence.
Confidence boosting is a vital and sometimes missing ingredient in many maths classrooms. As HoD I always elect to be timetabled with the lower ability groups because one of my prime concerns is the stream of students who will walk into the department that first week in September and say, ‘I’m rubbish at maths.’ Sometimes it is interesting to explore where this mindset has come from, but from the first minute in the classroom the primary objective is to reverse it.
We attempt to measure progress in a variety of ways. End of term reports sometimes end up being lists of achievement, and this has its place, but other mentions about attitudinal or emotional challenges met and mastered are equally important.
For me the autumn term is more about whether students end up at Christmas with a changed attitude, a raised level of confidence and the realisation that maths isn’t hard, that it does matter and they needn’t by anxious about walking through my door. Put these things in a report and, together with the change of heart about maths visible in their children, maybe parents will also start to realise that maths isn’t hard.
May 1, 2015 / cpstobart / 0 Comments
Started a conversation with Julia at Revision Buddies about planning the revision of the cool maths GCSE revision app that I had provided content for. If you have followed the blogs here and the ones I wrote for the Collins Online Maths Festival last month you will have an appreciation of the changes that are coming.
Watch this space!
